________________________________________________________

________________________________________________________

It is important to note that Edgeworth's claims about "indeterminacy" of exchange depend very much on his vision of exchange. Edgeworth adhered strictly to the idea that the exchange process that went on in reality was neatly captured by his "recontracting" logic. But it is not the only logic. Could Jevons be right? Could barter exchange be determinate if we consider other ways by which the exchange process is conducted?

Certainly, Edgeworth's contemporaries -- and many others since -- disagreed with him. At the simplest level, they did not believe that Edgeworth's recontracting process was actually "realistic" or, at least, that it was incomplete. This is because there remains the question of bargaining over the core allocations. Relative bargaining power -- persuasive ability, patience, force of character, attitude towards risk, etc. -- might be brought in to play and make the outcome a little more certain. For instance, if Friday has less "persuasive power" than Robinson, then it is more likely that he will end up at a core allocation closer to his autarky indifference curve. Bargaining theory, as pursued by Frederik Zeuthen (1930) and John Nash (1950, 1953), employs precisely this line of reasoning to argue that there is a (unique) core allocation that will result when we take a richer view of bargaining.

Edgeworth, while acknowledging the role of "dissimulation and objectionable arts of higgling" (Edgeworth, 1881: p.30) in determining which of the final settlements was actually chosen, nonetheless felt that these bargaining issues were outside the sphere of economics. His preferred method of resolving the indeterminacy question was via ethics, in particular contractarian utilitarianism.  

(2) The Utilitarian Solution

It is important to note how Edgeworth's moral sense was offended by the "Art of Bargaining -- higgling dodges and designing obstinacy, and other incalculable and often disreputable accidents." (1881: p.46). He may have found indeterminacy of contract, but he was not happy about it. Indeterminacy means that the final position in the core is uncertain; it will call forth all the horrible arts of face-to-face bargaining. Where the trade finally ends up will be "a throw of a die loaded with villainy" (1881: p.50).

It was Edgeworth's ethical outrage at bargaining that led him to conclude that "[t]he whole creation groans and yearns, desiderating a principle of arbitration, an end of strifes" (1881: p.51). His preferred principle of arbitration was the utilitarian solution: let us choose the allocation in the core that maximizes the sum of individual utilities. Despite his invention of indifference curves, Edgeworth had not gone the next step to renounce interpersonal comparisons of utility. Yet his justification for using the utilitarian calculus, as intimated, was based on the idea of uncertainty. As the final position in the core is uncertain, so the parties will (or at least ought to) naturally opt for the utilitarian middle.

He had already laid out his ethical utilitarian doctrine in earlier books and articles (e.g. Edgeworth, 1877, 1879). But he found its most fortuitous application in his 1881 indeterminacy of barter result. As Peter Newman notes, "Edgeworth's attempt to move from his "weak" bargaining solution, the [core], to a "strong" solution, the utilitarian point, cannot be judged a success. But what a road travelled for such an Oz-like destination!" (Newman, 1987).

Price-mediated exchange theory, what we have called "Walrasian exchange", asserts that, given a set of prices by the market, agents evaluate their budgets and then choose the bundle which maximizes their utility over them. That bundle constitutes their demand for goods. Under certain conditions on preferences (strict convexity, etc.) this demand will effectively constitute a specific offer to trade, but these offers are made "to the market" and not to any particular person. Unlike the Edgeworthian exchange process, a proposed trade in a Walrasian context does not specify explicitly "what for what and with whom". The proposed trades take the form of "demands" from the market and "supplies" to the market. Walrasian traders are thus relatively more passive and anonymous than Edgeworthian traders. For Walrasians, market prices contain all the necessary information about trading possibilities.

Now, the rules of Walrasian exchange are such that these trading offers will only be fulfilled if the market deems that the offers match. If they do not, then everything is canceled and the market will provide a new set of prices and ask the traders to determine their demands and supplies again. An "equilibrium" (prefixed "general" or "market" or "Walrasian" or "competitive") arises when total demands match total supplies in each market.

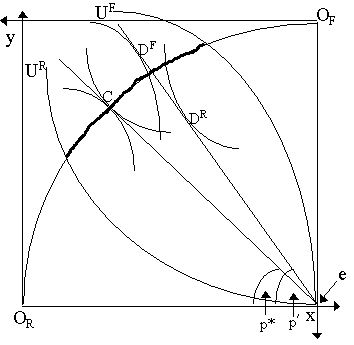
The relationship between the Edgeworthian core and the Walrasian equilibrium is quite tricky, and the insights Edgeworth (1881) brought into this story are quite remarkable. The first result is simple: that the Walrasian equilibrium will be somewhere in the Edgeworthian core. This is almost self-evident intuitively: as we saw in our discussion of Walrasian exchange (and the Paretian system), the Walrasian equilibrium will be at the tangency of indifference curves, somewhere in the inside the "lens" formed by the autarky indifference curves. This is shown in Figure 1. At prices p* (a vector), the respective budget constraints are formed (relative to the origins O_F and O_R). Notice that the bundles chosen by Robinson and Friday -- at the tangency of the budget line p* and their highest indifference curves -- will be C. This implies that the demands and supplies match each other perfectly: at C, the amount of good y supplied by Friday matches the demand for good y by Robinson, while the amount of x supplied by Robinson matches the demand for good x by Friday. Thus, C represents a Walrasian equilibrium allocation.

Fig. 1 - Walrasian Equilibrium and Disequilibrium

At a different set of prices, say p¢ , the budget constraints will be different. Notice in Figure 1 that now Robinson chooses bundle D^R and Friday chooses budle D^F. The demands and supplies do not match this time: Friday demands more of good x than Robinson is willing to supply, while Robinson demands less of good y than Friday has supplied. This is a Walrasian "disequilibrium". Trade cannot happen under prices p¢ .

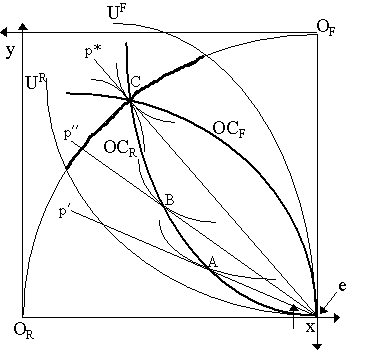
It is important to note that at the Walrasian equilibrium, the allocation C is in the Edgeworthian core (denoted by the thick portion of the contract curve). Why this is so is self-evident: in equilibrium, indifference curves are tangent to each other at the same point. This only happens on the contract curve -- and, as we saw earlier, for a two-person economy, the portion of the contract curve within the lens is the Edgeworthian core.

Edgeworth depicted the Walrasian equilibrium in the core by the use of what were later labelled "offer curves" or "price-consumption curves" in his net trade diagram (Edgeworth, 1881: p.114). An offer curve depicts the locus of demands for an agent at different prices. In Figure 2, we see that as we vary the price from p¢ to p¢ ¢ to p*, the demands of Robinson move from A to B to C. The locus tracing out these demands, Robinson's "offer curve", is denoted in Figure 2 by the curve OC_R. A similar exercise for Friday will yield Friday's offer curve, OC_F. Notice that the Walrasian equilibrium will be where the offer curves intersect -- namely, at point C in Figure 2.

Fig. 2 - Offer Curves

[Note: Offer curves were introduced by Alfred Marshall (1879), with a slightly different meaning as they incorporated production. Edgeworth's pure exchange offer curves were termed "offer curves" by W.E. Johnson (1913) and A.L. Bowley (1924). According to Newman (1965: p.104), Edgeworth did not approve of the term and used "trading curves" himself. We have referred to them as "price-consumption" curves elsewhere, but here shall retain the usage "offer curve".]

Of course, C is not necessarily the only Walrasian equilibrium. Walrasian equilibrium need not be unique. Despite his use of offer curves, Edgeworth himself recognized the possibility of multiple Walrasian equilibria (e.g. Edgeworth, 1881: p.46, fn.1). There might be another set of prices -- call it p** -- which also yields an equilibrium. Needless to say, this equilibrium will also be in the core. So, all Walrasian equilibria are in the core.

Proving this is elementary. Let us define a Walrasian equilibrium:

Walrasian Equilibrium (1): A price-allocation pair, (p, {x^h}_{hÎ H}) is defined as a "Walrasian equilibrium" if the the following is true for every household h Î H:

(i) x^h Î argmax u^h(z) s.t. pz £ pe^h for all h Î H

(ii) å _{hÎ H} x^h = å _{hÎ H}e^h

where condition (i) states that every household receives what it demands (note that x^h is the demand of the hth household, i.e. the bundle which maximizes the hth household's utility subject to his budget constraint) and (ii) all markets clear. An alternative definition can be offered:

Walrasian Equilibrium (2): A price-allocation pair (p, {x^h}_{hÎ H} ) is defined as a "Walrasian equilibrium" if, for every h Î H, y >_h x^h implies py > pe^h.

i.e. if anyone strictly prefers another bundle (y) to their Walrasian allocation (x^h), then that bundle is necessarily unaffordable, i.e.py > pe^h means it is outside the hth household's budget constraint.

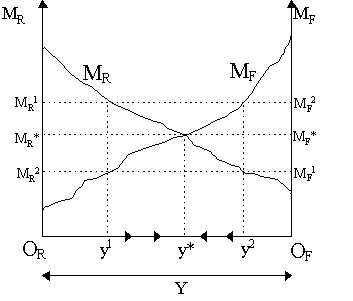
We denote the set of Walrasian equilibria in an economy as W(E). We now turn to the proposition we are interested in (Debreu and Scarf (1963) credit Lloyd Shapley for this proof):

No less important, Edgeworth noted that many of the points in the core are not Walrasian equilibrium allocations. Examples of this are easy to construct, and we leave it up to the reader to come up with some explicit examples. We will just refer to Figures 1 and 2 to illustrate this.

Alfred Marshall (1890: App. F), although a utilitarian himself, did not feel that one needed to go to Edgeworth's philosophical lengths to resolve the indeterminacy problem. In fact, he believed it resolved itself. Marshall agreed, in principle, with Edgeworth's indeterminacy of barter, yet nonetheless felt that determinacy would be "restored" in practice, i.e. that an actual process of barter exchange would ultimately end up at a unique, equilibrium trade.

Marshall's argument rested on two interesting pillars. Firstly, he viewed the barter question quite differently from Edgeworth. For Marshall, contracts are binding and not merely hypothetical. In other words, the "exchange process" for Marshall is a process of actual exchanges, not the process of suggestions and counter-suggestions mapping out the competitive field that we find in Edgeworth. Much of the subsequent miscommunications between Marshall and Edgeworth rest in good part on Marshall's failure to appreciate the purely suggestive nature of Edgeworthian contracts. The second pillar, to which Marshall attached the highest importance, was the assumption of a "constant marginal utility of money" -- what has become known as Marshall's postulate..

We can reconstruct Marshall's argument in the following manner (cf. Newman, 1965; Walker, 1969). Let us presume that Friday and Crusoe are merely trying to determine the amount of good y that Friday will give to Crusoe. Notice that we are ignoring what Crusoe gives to Friday, namely good x. Let the total amount of good y be denoted Y. Consider now Figure 3. The length of the horizontal axis is precisely Y. The origin O_R measures the amount of good y that Robinson receives, while the other origin, O_F measures the amount of good y that Friday surrenders.

For the moment, let the curves M_R and M_F denote the marginal utility of good y for Robinson and Friday respectively. By the principle of diminishing marginal utility, then the curve M_R shows how the marginal utility of good y diminishes for Robinson the greater the amount of y he commands from Friday. Similarly, the marginal utility of good y for Friday, M_F, increases the more y he gives up to Robinson (and the less he keeps for himself). These curves intersect at the amount y*, where, it is important to note, the marginal utilities of both Friday and Robinson are equal.

Fig. 3 - Marshallian Barter

 Suppose that Robinson acquires good y from Friday with "money" (good x). Now, suppose we begin at position y₁, where Robinson's marginal utility for good y (M_R¹) exceeds Friday's (M_F¹). This implies that the money Robinson is willing to give Friday for an extra unit of y is greater than the money Friday would demand from Robinson to yield up a unit of y. Consequently, there is scope for trade: Friday will give good y to Robinson for some amount of money, the exact price being somewhere between M_F¹ and M_R¹. This will bring the allocation of y closer to O_F and farther from O_R.

They will continue to trade until they reach y*, where M_F* = M_R*, the marginal utilities are equal. In other words, there is no incentive to continue trading further. Now, naturally, they may overshoot with their trades and, say, end up at y₂. At this point, the marginal utility of good y for Robinson (M_R²) is lower than the marginal utility of good y for Friday (M_F²) -- thus it is in both their interests if Robinson were to sell some of good y back to Friday.

The main point of this Marshallian process is that the trade y* forms a unique point of gravitation for the exchange between Robinson and Friday. One way or another, there is a unique y* which forms the endpoint of barter trade. Several conditions must be met for the analysis in Figure 3 to be reasonable. Firstly, we would like the curves to intersect somewhere in the middle; if they do not, then y* will be on one of the corners, either O_R or O_F (such a corner solution is not really a problem, though). Secondly, we would like it that trade be continuous, so that we avoid overshooting which might result in, say, the creation of a limit cycle (e.g. y¹ to y² back to y¹ and then y², etc.), so that we fluctuate around y* without ever approaching y*.

The third and most important condition is that the marginal utility of money is constant -- Marshall's postulate. We see why this is important when we realize that M_R and M_F are not really the "marginal utility of y" curves, but rather the marginal rates of substitution of good y for money, it is the ratio of marginal utility of good y to the marginal utility of money, i.e.

M_R = (¶ U^R/¶ y)/(¶ U^R/¶ x) = MRS_yx^R

M_F = (¶ U^F/¶ y)/(¶ U^F/¶ x) = MRS_yx^F

where ¶ Uⁱ/¶ y is agent i's marginal utility of good y and ¶ Uⁱ/¶ x is his marginal utility of money (x is money). Now, if the marginal utility of money is constant for both agents -- i.e. it does not diminish or increase for either of them -- then the shape the M_R and M_F curves takes is indeed the governed solely by the diminishing marginal utility of good y, and the rest of the analysis proceeds as we had it above.

However, if the marginal utility of money is not constant, then we cannot be sure of this as the willingness to acquire good y must be balanced by the willingness to surrender money. Trading would "end" when the marginal rate of substitution of one agent would be equal to the other's, but that "end" would not be unique -- "an equilibrium would have been reached; but it would be an arbitrary equilibrium." (Marshall, 1890: p.653). This is Edgeworth's indeterminacy in barter. But if we assume a constant marginal utility of money, then we would converge to a determinate trade, such as we have in Figure 3.

Analytically, we obtain constant marginal utility of money if we have separably additive utility functions -- like the ones Marshall used -- where:

U^R(y, x) = V^R(y) + a x

U^F(y, x) = V^F(y) + b x

where V^R(·) and V^F(·) are the regular concave utilities from good y while a and b , attached to money (good x), are constants. Notice that the marginal rates of substitution are therefore

M_R = (¶ V^R/¶ y)/a

M_F = (¶ V^F/¶ y)/b

for Robinson and Friday respectively. Notice that the marginal rates of substitition are independent of the quantity of money, x but depend only on y. By diminishing marginal utility with respect to y, we know that therefore:

¶ M_R/¶ y = (1/a )·¶ ²V^R/dy² < 0

¶ M_F/¶ y = (1/b )·¶ ²V^F/dy² < 0

so the marginal utility curves are monotonically downward-sloping. This is exactly what we see in Figure 3. Thus, the tangency of indifference curves -- W.S. Jevons's (1871: p.95) condition for equilibrium in barter exchange -- implies that M_R = M_F, which is true only at the unique point y* in Figure 3.

In terms of Edgeworth-Bowley boxes, the constant marginal utility of money assumption implies that the core portion of the contract curve is a straight line. This is shown in Figure 4. In other words, the indifference curves of Robinson and Friday are always tangent whenever y* is traded -- regardless of the corresponding amount of money, x. Thus, in Figure 4, both C and D are solution trades -- both correspond to the position y* in Figure 3 (adjusted for endowment). Notice that this implies that at y¹, then necessarily Robinson's indifference curve is flatter than Friday's (cf. point F), which translates back to saying that M_R > M_F at y¹. Conversely, at level y², Friday's indifference curve is flatter than Robinson's, thus M_R < M_F, as we had it previously. Thus, the situation depicted in the box in Figure 4 corresponds (roughly) to the equivalent situation depicted in Figure 3.

Fig. 4 - Marshall's Postulate

We see that Alfred Marshall's claim that there is a unique solution, a unique amount of good y traded, in barter exchange relies heavily on the assumption of constant marginal utility of money (or, more generally, constant marginal utility of good x -- which may be money or something else). This is, effectively, a restriction on preferences which is not really justifiable. And Marshall agreed that this was a very special case. But Marshall also convinced himself that if the amount of good x (i.e. money) is so large and well-distributed so that its marginal utility is miniscule for all traders, then its marginal utility will be "practically constant". Now, in many cases of barter, he agrees, this will not be true -- and thus the equilibrium will be indeterminate, precisely as Edgeworth demonstrated. However, he argued that there are many cases, in well-organized advanced economies, where this is the case, and the equilibrium is unique.

This argument, of course, was brought in without evidence. It is quintessential Marshallian fudging. However, it was important for Alfred Marshall for he believed that his price-mediated exchange theory -- i.e. demand-and-supply theory -- had to be defended from the Edgeworthian critique at all costs. After all, price-mediated exchange focuses on a determinate result, that of equilibrium between demand and supply functions. If, as Edgeworth asserts, the market solution is only one of many possible solutions (at least in any situation that is less than perfectly competitive), then Marshall's demand-and-supply theory could be rendered "practically" useless. So, by arguing that the marginal utility of money was "practically" constant, then Marshall restores determinacy of exchange and side-steps Edgeworth's critique, or rather confines it to those "rare" cases of pure barter exchange with non-constant marginal utilities of money. As he notes:

"The real distinction then between the theory of buying and selling [i.e. demand-and-supply] and that of barter is that in the former it generally is, and in the latter it generally is not, right to assume that the stock of one of the things which is in the market and ready to be exchanged for the other is very large and in many hands; and that therefore its marginal utility is practically constant." (Marshall, 1890: p.654).

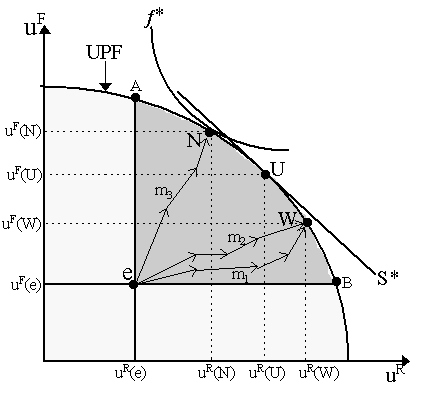
Edgeworth was not fooled, of course. He realized that it was Marshall's theory, and not his own, which required an "impractical" assumption. Edgeworth's (1891) gentle response to Marshall and his hackman, Arthur Berry, demonstrated that Marshall's postulate implied exactly the very special result we see in Figure 4 -- namely, that the contract curve is a line parallel to one of the axis -- and that x remains indeterminate. He also argued, more generally, that any incremental barter process of actual exchanges, far from driving the economy to the unique equilibrium, would merely drive it to one of the core allocation points, an arbitrary result.

Edgeworth's criticism (and his accompanying diagram) were partly incorporated in later editions of Marshall Principles (Note XIIbis, p.695-96) but they did not lead Marshall to change the substance of his argument. For a more detailed review of the Marshall-Edgeworth barter controversy, see Creedy (1986) and Newman (1990).

The various sorts of determinacy we have run over can be compared by depicting them all within the utility possibilities frontier (UPF) in Figure 5. The lightly shaded area below the UPF plots the different levels of utility attainable by Robinson and Friday within their pure exchange economy. Thus, this corresponds to the utility combinations achievable in the entire area of the Edgeworth-Bowley box. The utility-combination achieved at the endowment is represented in Figure 5 by e, so the autarky utility levels are represented by u^F(e) and u^R(e). The utility combinations attained in the area of the "lens" formed by the autarky indifference curves in the box diagram is represented in the UPF by the darkly shaded area in Figure 5.

The UPF itself corresponds to utility combinations achieved in the box's contract curve. The Edgeworthian core is represented by the curve connecting points A and B. Where on the core we end up, as we know, is indeterminate -- thus any of the utility combinations on this segment are feasible. The utility-combination achieved at the Walrasian equilibrium is represented in Figure 5 by the point W -- which, as we can see, is on the boundary, and thus in the core. This is the "Walrasian" equilibrium solution.

Edgeworth's utilitarian solution is denoted U in Figure 5, and is obtained by maximizing a utilitarian social welfare function, S, where:

S = å _h=1^H a ^hu^h

i.e. the weighted sum of utilities (in our special case, the weighted sum of Robinson's and Friday's utility levels). This utilitarian social welfare function will define a set of linear "social indifference curves", its upper contour set. The highest social indifference curve, S*, represents the maximimum social utility obtainable in the economy. Thus, point U in Figure 5 represents the utility-combination that maximizes social welfare. As we see, U corresponds to some allocation in the core.

[Note: It is not necessarily true that the utilitarian solution will be in the core. The utilitarian solution will definitely be on the contract curve -- represented by the entire UPF -- but its exact location could actually be outside the core, e.g. on a UPF point to the left of point Aor to the right of point B in Figure 5. Edgeworth did not seriously consider this possibility and insisted that U lies in the core portion of the UPF. This would certainly be true if the utilitarian solution were not applied to the whole field of trade, but, rather, limited only to the darkly shaded area in Figure 5, i.e. if we applied the utilitarian solution to utility gains, rather than utility levels. However, Edgeworth did not argue this.]

Fig. 5 - Determinacy Compared

Notice that the utilitarian solution (U) does not necessarily coincide with the Walrasian equilibrium (W) solution. Both are in the core, but at different points in the core. This means, as Edgeworth was wont to insist, that the market does not (necessarily) lead us to the highest social welfare. In this respect, Edgeworth was in agreement with Léon Walras (1874) and Knut Wicksell (1893) (see our discussion of Paretian social welfare): they did not believe that the free play of the market will yield what good ethics recommends. It can perhaps be argued that it was precisely Edgeworth's belief in the non-ethical outcome of the market that accounts, at least partly, for his general hostility to the ethically-loaded marginal productivity theory of distribution. (cf. Edgeworth, 1904).

An interesting point in Figure 5 is the "Nash Bargaining Solution" which we have denoted as N. This arises from the tangency of the UPF and the Nash bargaining locus, ¦ *, which can be seen as a sort of convex social indifference curve that captures the various aspects of bilateral bargaining which Edgeworth avoided. This would be, according to John Nash (1950), the solution achieved by a pair of bargainers. Note that, again, it is not the same as the utilitarian solution nor the Walrasian equilibrium. Yet this, as Nash demonstrated, would solve the indeterminacy problem. It was precisely this road that Edgeworth refused to take, on ethical grounds.

Finally, let us consider how Marshall's (1890: App. F) solution could be depicted. Effectively, Marshall argued that starting from the endowment point, e, we would "gradually" approach the Walrasian equilibrium by a series of (real) barter trades. This is represented in Figure 5 by the paths m₁ and m₂, emanating from the endowment point e and approaching the Walrasian equilibrium W. However, Edgeworth's (1891) criticism of Marshall was that it was not necessarily true that every path of actual barter exchanges emanating from e would end up at W. He pointed out that there were other plausible paths, such as m₃ in Figure 5, which end up at different points in the core (in this case N).

[Note: it is interesting to note that when we consider the various "nodes" (or "rounds of trade") through which the Marshallian barter path takes (roughly represented in Figure 5 by the arrow-heads along the paths), that there is an implicit restriction that we can only move in a northeasterly direction from any node (otherwise it would not be utility-improving for both agents). So, if the traders start on a path which begins like m₃, whose first node (i.e. the result of the first round of trade) is to the northwest of W, they can actually never get back to W, because that would require a southeasterly move -- i.e. a move in which one of the agents (in this case Friday) loses utility.]