|
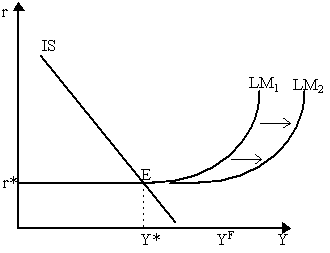
Does the Neo-Keynesian model always rely on sticky wages to create unemployment? Not quite. At least two other possibilities without sticky wages are immediately observable. The first was identified by John Hicks as the "special form of Mr. Keynes's theory" (Hicks, 1937: p.109) - namely, the "liquidity trap". As Hicks originally expressed it, the "special form" was when the income elasticity of money demand was near-zero. Later on, the liquidity trap became identified as the case when interest elasticity of money demand was near-infinite. Whichever the case, they both amount to the same thing: namely, the LM curve is basically flat. Naturally, it may seem ludicrous to presume such an extremity for the entire LM curve, but what was suggested is that it is conceivable that the liquidity trap emerges at very low levels of interest. Thus, the LM curve has a flat portion at low levels of output and interest - as shown in the Figure 5. This, it was claimed, was the case because if interest rates were very low, people would expect interest rates to rise in the future and thus would be willing to hold any extra amount of money made available in the interim in anticipation of that rise (i.e. there is no point in buying bonds at low interest rates/high bond prices if one expects interest rates to rise/bond prices to fall).
The economy is in a "liquidity trap" if the IS curve intersects the LM curve somewhere in this flat portion. In this case, falls in money wages may push the LM curve to the right (in our Figure 5, from LM1 to LM2), but the equilibrium level of output Y* and equilibrium interest rate, r* will remain virtually unchanged and we move nowhere nearer the full employment output level, YF. In short, the "Keynes effect" will be disabled. This was, incidentally, the only other "Keynesian" case allowed by Modigliani (1944). A second possible case, as stressed by James Tobin (1947), is if the investment demand function is interest-inelastic. In this case, the IS curve would be quite steep - in the extreme, completely vertical - so that a rightward shift in the LM curve due to the Keynes effect can lower interest rates all it wishes, but investment and hence aggregate demand, equilibrium output Y* and employment will remain unchanged. Sticky money wages, liquidity traps and insensitive investment functions were the three rallying points for the Neoclassical-Keynesian Synthesis. Effectively, they turned the Keynesian revolution on its head by concluding that Keynes's theory was merely the "special" case of a more general Neoclassical one - true when any of these three conditions obtained, untrue otherwise. They did not worry too much that they had, in this manner, effectively eliminated the theoretical significance of Keynes's General Theory. After all, they argued, Keynesian theory still had "practical" significance: these three special cases were quite plausible in the "real world" and thus "Keynesian" analysis was still relevant. Nonetheless, all was not well for long. Specifically, Gottfried Haberler (1937), Tibor Scitovsky (1941) and Arthur C. Pigou (1941, 1943, 1947) postulated that the consumption decision is based not only on current income but on "real net wealth". Initially, "real net wealth" referred to the real supply of money (M/p) and the real supply of bonds (B/p). The conventional Keynesian consumption function makes consumption, at best, a function of real disposable income and interest rates, but Haberler-Pigou proposed the inclusion of real net wealth as well, thus C = C(Y, r, V) where V = M/p + B/p. Lloyd Metzler (1951) subsequently argued for the inclusion of capital (K) as a component of "real net wealth" - even if Keynesian theory disregarded it in simple IS-LM equations, that only implied that it was assuming that bonds and capital were perfect substitutes. Thus, we can let V = M/p + B/p + K denote "real net wealth". The issue of why consumption is related to real net wealth is controversial. The proposition is not, strictly speaking, that agents' "consume" out of wealth (for that would require the sale of wealth to somebody else). Rather, it is a sort of "feel-good" relationship: people with large amounts of wealth are "richer" and thus "feel" as if they can consume more out of current income. At least one economist, Lerner (1973), questioned this reasoning, and asked mischievously to allow it to go the other way - say, a "feel-bad" relationship between wealth and consumption (particularly if B was composed of government bonds which people may believe they have to pay back later in taxes). The implication of this new consumption function should be clear. In situations of unemployment, as money wages and price levels decline, then the real money supply rises (the Keynes effect) which, as we saw, shifts the LM curve to the right. However, the "Pigou Effect" (or "Real Balance" effect) implies that as M/p rises, so does V and consequently consumption rises as well - shifting the IS curve to the right. Thus, Pigou (1943) proposed, even the "special cases" of a liquidity trap or interest-insensitive investment are not sufficient to maintain unemployment equilibrium as the rightward shifts of the IS curve via the "Pigou Effect" will ensure we are taken to full employment equilibrium. Thus, the only possible way to have unemployment equilibrium in a Keynesian model is if there are sticky wages and prices, period. While many Neoclassicals cheered this development, there was a sense of unease about these wealth effects for the implications they had for their own macroeconomic theory. Specifically, as Lloyd Metzler noted:
In other words, the "dichotomy" between real and monetary sectors, so cherished by Neoclassicals, was broken by the Pigou Effect as, apparently, increases in the money supply could now affect real items like consumption, interest and output. Was neutrality demolished? In a careful and elaborate disquisition and elucidation, Don Patinkin (1948, 1951, 1956) arrayed various arguments in defense of this "wealth effect". Specifically, he noted, the Neoclassical theory was contradictory anyway - it is impossible to reconcile the Quantity Theory of Money with the assumption of dichotomy. In fact, as he went on to argue, the "neutrality hypothesis" and the Quantity Theory itself requires a real balance effect that violates dichotomy. Furthermore, it helps solve the old problem of negative interest rates that the Neoclassical loanable funds theory could not really rule out. Following, Metzler (1951), there are also some additional comments on the impact of prices on output. Let us push the analysis one step further and consider allocation between liquid assets (money) and illiquid assets (capital). If price levels decline (in unemployment, etc.), real wealth increases, but there is also a liquidity effect because the proportion of wealth made up of liquid money balances as opposed to illiquid capital increases. Thus, agents have excess liquidity and will try to get rid of it by decreasing their money demand. Thus, there can be an additional shift in the LM curve. Metzler's analysis also led to discussions about fiscal and monetary policy - specifically, the manner by which government increases money supply or how it finances spending will have substantial effects on the resulting outcomes via these wealth effects. The analysis of J.G. Gurley and E.S. Shaw (1960) and the famous fiscal policy work of Alan Blinder and Robert Solow (1973) follow Metzler's guidelines in this regard. However, problems quickly arose. The first was Michal Kalecki's (1944) reminder about the components of net wealth: specifically, he noted, only "outside" money and "outside" bonds can constitute net wealth in macroeconomic analysis. To use the terminology of Gurley and Shaw (1960), "inside" money (i.e. bank deposits) and "inside" bonds (private sector debt) cannot be considered part of the net wealth of the economy because every person's asset is another person's liability so that, upon aggregation, these inside debts will cancel out. Thus, if the Pigou Effect is to work, it must work on the narrow components of "outside money" (high-powered money, i.e. currency and Central Bank reserves) and "outside bonds" (i.e. government-issued bonds and bills) and real capital - presumably, the only assets without a corresponding liability in aggregate. Effectively, Kalecki's qualification effectively reduced at least the empirical importance of the Pigou Effect - a fear also expressed by Pigou (1947) and Patinkin (1948). Don Patinkin (1956, 1972) seemed to agree that outside wealth should be regarded only as the liabilities of the government and real capital. However, Boris Pesek and T.R. Saving (1967) disputed this conclusion and argued that inside money is part of the payments system and thus it provides some degree of utility from the "services" it provides. "Money yields income to the owner without yielding a negative income to the producer of it or to anyone else. Consequently, money must be a part of the net wealth of the community." (Pesek and Saving, 1967: p.246). These "services", then, ought to imply that at least some forms of inside money ought to be considered "net wealth" in the aggregate. In Pesek and Saving's view, inside money should be regarded as part of net wealth provided no interest is paid on that portion. This is echoed by Harry Johnson (1969) who argues further that it does not even matter that interest is not paid on deposits - as long as the interest gains on money do not exceed that on alternative assets (e.g. bonds). The demand curve for money, he argues, is like any demand curve: there is a consumer surplus. This means that for any given level of interest, there will be some amount of money which would have been held anyway at a higher interest. Both Pesek and Saving (1967) and Johnson (1969) argue that the Kalecki-Gurley-Shaw definition of net wealth is inadequate and that net wealth should be wider. The question of public bonds has fed an even more incendiary fire. Famously, Robert Barro (1974) attacked the notion of government bonds being part of net wealth. In what has been later dubbed the "Ricardian Equivalence Hypothesis", Barro argued that by issuing bonds as a method of debt-financing, the government is merely postponing the taxation required to repay them. If households regard consumption decisions intertemporally, they will not consider government bonds as "outside" since any current windfall gains will be entirely offset by future expected taxation. Government bonds, according to Barro (1974), should therefore not be included as part of net wealth - thereby diminishing the power of the Pigou Effect even further. Others have since disputed the assumptions underlying Barro's "Ricardian Equivalence Hypothesis" (e.g. Tobin, 1980), which we discuss more fully elsewhere.
|
All rights reserved, Gonšalo L. Fonseca