|
Contents (A) "Crowding Out" and "Crowding In"
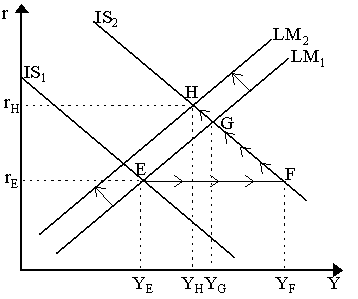
Abba Lerner's "functional finance" had been so effective in dismissing the deficit bugaboo that, throughout much of the post-war period, most analysis of public policy ignored the impact of the resulting debt. However, the ascendancy of the LM side of the Keynesian model re-opened the deficit debates in the late 1960s and early 1970s. Obviously, if money, bonds, etc. have strong impacts on the economy, the manner in which the government decides to finance its spending decisions will be of instrumental importance. (A) "Crowding Out" and "Crowding In" One of the crucial terms in the lexicon of the new deficit debates of the 1970s was the "crowding out" effects of deficit-financed government expenditures. We can differentiate between two aspects of this concern: (1) that government spending "crowds out" private spending by competing for scarce resources - what can be termed "real" crowding out; (2) that government borrowing "crowds out" private borrowing by raising interest rates - what can be termed "financial" crowding out. Juxtaposed to this this are real and financial "crowding in", i.e. situations where deficit-financed government expenditures increase private expenditures and private borrowing. The notion that bond-financed government spending "crowds out" private borrowing and hence expenditure, worried early critics of deficit-spending. In the Neoclassical loanable funds theory, bond-financed government deficits compete with private investment for savings - thereby raising interest rates and thus the cost of capital for private borrowers which may make at least some of them leave the loan market. However, in the Keynesian view, such an argument could not be made as there is not a fixed "stock" of savings in any sense of the term. By the Keynesian multiplier, deficit-financed government spending will lead to a rise in output which will generate the very savings necessary to back it up. Thus, in the Keynesian model, private sector borrowing is not "crowded out" by government borrowing. However, the Keynesian model does allow for a small measure of financial "crowding out", but of a quite different nature. Firstly, as output rises, the demand for money rises and that can raise interest rates somewhat. We shall call this the "money demand effect". Secondly, by supplying government bonds, the economy-wide supply of liquidity, which can be captured by the money-bond ratio, has decreased. As a result, people will require a higher interest rate to hold this more illiquid position. We shall call this the "portfolio effect". We can see what the full impact of a bond-financed expansion in government spending would be in a Keynesian context by examining Figure 10. From an initial starting point at E, the fiscal expansion implies that the IS curve would shift to the right substantially which would imply a horizontal rise in output (YE to YF) which would then be followed, from, the money demand effect outlined above, by a rise in interest rates and thus a fall in investment and a fall in output back to YG (i.e. we climb up the LM curve to the equilibrium G). However, the "portfolio" effect of the extra supply of bonds would imply that there is also a slight shift leftwards of the LM curve (LM1 to LM2) so that the new equilibrium output is actually YH and the interest is rH. Consequently, there is some degree of decreased investment due to increased government spending - the exact magnitude of this "crowding out" depending upon the income and interest elasticities of money demand and investment.
The impact of this form of crowding out on private investment may be diverted via domestic exports. Under a flexible exchange regime, increased budget deficits place upward pressure on interest rates, which may lead to higher capital inflows and thus an appreciation of the country's currency. This, in turn, reduces net exports. Thus, in this manner, government deficits would "crowd out" net foreign investment rather than domestic investment. There are two alternative forms of financing government expenditure: by raising taxes or printing money. Both can yield some degree of "crowding out" via the money-demand effect. Whatever the form of financing, any rise in output will raise the transactions demand for money and thus increase interest rates and lower investment (a movement along the LM curve). However, a tax-financed fiscal expansion would lead to a relatively more severe real "crowding out" of private expenditure as consumption would fall due to the lower disposable income from increased taxation - thus the IS curve would shift back down. However, the celebrated "balanced budget multiplier theorem" of Haavelmo (1945) shows that an equal increase in government expenditure and taxation would lead to a net increase in output as the multiplier effect of government spending is greater than the fall in output implied by an equivalent increase in taxation. We can see this directly from our equations. Suppose there is an increase in government spending and an increase in autonomous taxation by exactly the same amounts, so that D G0 = D TX0, so the government budget remains in the same balance as before. However, government expenditure has a direct impact on aggregate demand while taxation only works indirectly through consumption, thus only a proportion of the increase in taxation actually impacts aggregate demand, specifically, cD TX0, where c is the marginal propensity to consume. As 0 < c < 1, then obviously D G0 > cD TX0 and so, from the multiplier equation, we see that:
Thus, equilibrium output increases even though the budget is balanced. Thus, tax-financed increases in government spending are, on net, expansionary, even though there is real crowding out. A money-financed increase in government spending has far more expansionary power than either bond-financed or tax-financed. The original government expansion increases output and, by the transactions demand for money, interest rates (IS shift right). However, the concurrent increase in money supply to finance this move will lower interest rates and increase output even more (LM shift right). The end impact on interest rates is ambiguous: in principle, there is no reason to assume a priori that the resulting interest rates will be higher or lower than the initial interest rates. Consequently, the net effect may still be a rise in interest rates - so there we would have at least a bit of the "crowding out" of investment via the money demand effect. However, it could also be that interest rates are lowered in the end, so that there is, in fact, a "crowding in" of investment from the money demand effect. In more careful models, there is also a "crowding in" version of the portfolio effect, identified by Benjamin Friedman (1978). Suppose we have a more detailed LM side with three assets (money, bonds, equity, or M, B and E, respectively). The excess bond supply that results from deficit-financing could be disposed of by agents moving into equity rather than money, thereby raising the price of equity and lowering its return. This last effect would, in turn, induce more private investment. Thus, in this case, there is a portfolio "crowding in". To understand this, consider the following. James Tobin (1961, 1969, 1982) had suggested that the LM portfolio approach should rely on considerations of the "relative substitutability" of assets. Simple Keynesian liquidity preference theory argues that money is contraposed to other illiquid assets (bonds and equity), thus implying that bonds and equity must be close substitutes. Hence, we can consider "liquid" assets = M and "illiquid" = B+E. Thus, if agents wish to keep a liquid/illiquid ratio (or M/(B+E)) of 50/50 in their portfolios, then if government bond supply increases due to deficit-spending, then illiquidity increases (tilting the ratio to, say, 40/60) which will induce an increased demand for money as people attempt to return to the 50/50 ratio they had before. As people move out of the illiquid bonds/equity complex into liquid money, this will raise the rates of return on both bonds and equity. Tobin suggests an alternative scenario where bonds are considered "liquid" and are thus closer substitutes to money than they are to equity. In short, "liquid" = M+B and "illiquid" = E so the liquid/illiquid ratio is now (M+B)/E. In this case, if the supply of government bonds increases, the "liquid" portion increases to 60/40 - inducing, therefore, an increased demand for equity in an attempt to re-establish the 50/50 portfolio. For asset market equilibrium, i.e. for people to accept the new 60/40 composition, there must be a fall in the rate of return on equity (but also a rise in the interest on bonds). Thus, Benjamin Friedman (1978) and other economists have argued for a "crowding-in" effect of government deficits on the basis of this second argument, i.e. that equity and government bonds are relatively poor substitutes (and bonds and money are relatively good substitutes). If we were to expand our asset menus to include short-term bonds, long-term bonds and capital, then we could also obtain a story of the impact of fiscal policy on the term structure of interest rates (e.g. Turnovsky and Miller, 1984). Namely, if a bond-financed fiscal expansion is expected, people have expectations of higher interest rates as a whole - this would lead to people moving out of long-term bonds and into short-term bonds which would depress the prices of long-term and increase the prices of short-term bonds - or, in terms of rates of return, it would increase that of long-term bonds relative to short, thus steepening the yield curve. Thus bond-financed fiscal policy expansions could lead to lower short-term rates and higher long-term rates temporarily. One of the things highlighted during the early deficit debates - particularly by Evsey Domar (1944) - was the "long run" implications of the Keynesian system. The IS-LM is purely short-run as it assumes that capital is constant and it ignores the accumulation of debt. The extension of Keynes's theory to the "long-run" was the basic theme not only of Domar's work, but also in the Keynesian theories of growth and the business cycle of Roy Harrod (1936, 1939, 1949), John Hicks (1950), and indeed, the Cambridge Keynesians: Joan Robinson (1956, 1962), Nicholas Kaldor (1956, 1961) and Richard Goodwin (1951, 1967). [We should strongly remind ourselves that the famous growth model of Robert Solow (1956) and Trevor Swan (1956) does not count as an extension of Keynesian theory into the long-run as it is inherently and completely Neoclassical - with no money, no investment function, no unemployment, it has absolutely no Keynesian features. Nonetheless, we should note that attempts were made by James Tobin (1955, 1965), Frank Hahn (1960, 1961), Duncan Foley and Miguel Sidrauski (1971), Jerome Stein (1969, 1971) and Hugh Rose (1966, 1967) to integrate Keynesian features into Solowian growth models.] However, the "long-run IS-LM" questions that concerned the Neoclassical-Keynesian Synthesists is somewhat less ambitious than fully-fledged growth models as they still kept capital constant. However, as Robert Mundell (1965) was to remind everyone, the flow variables in a period must be added to the stock variables of the past - and these will affect the next "short run" equilibrium. In our case, the bond-financed deficit of today increases the accumulated public debt of tomorrow. How might this affect tomorrow's equilibrium? Furthermore, what happens to the deficit in the next period? The deficit may be smaller because the increased income associated with the deficit-induced output expansion will yield higher taxes, but it still may very well be there - in which case a new stock of bonds must be issued next period. In fact, as long as the deficit remains, there must be repeated issuing of new bonds which will lead to continual increases in the stock of public debt. What will be the impact of this accumulating debt on equilibrium output? The modest Neo-Keynesian question, then, was merely this: what is the consequence of a one-time increase in deficit-financed government expenditure in the longer-run? That flows (like the deficit) lead to continual increases in the stock of assets implies that a "long-run" equilibrium is reached only when the government's budget is balanced so that the accumulation of debt stocks ceases (or converges to a steady state). What sort of position will the economy be in when (and if) this finally happens? The analysis of this "long-run multiplier" was initiated by Carl Christ (1968). We begin from the simple government budget constraint in the short run is:
where G is government spending, T(Y) is taxes, iBB is debt service payments and dM/dt and dB/dt is money issue and bond issue respectively. The long-run constraint is obtained by integrating these terms over an infinite horizon and imposing the so-called "no-Ponzi game" condition that debt not increase faster than the interest rate. Doing so, we obtain the long-run government budget constraint:
where PV(T) is the present value of a future stream of taxes and PV(G) is the present value of a stream of government spending while B0 and M0 are the initial stocks of bond and money. The long-run government budget constraint implies that the present value of future surpluses (PV(T) - PV(G)) must be equal to the initial stock of bonds (B0) and money (M0). Thus, the constraint implies that the government which issues bonds (B0) must follow a expenditure/taxation path such that it can generate future surpluses of equal value, i.e. it must eventually be able to pay back the principal on its debt. Alan Blinder and Robert Solow (1973) used this notion to analyze the long-run implications of a one-time increase in government spending financed by bond issue. Other models which deal with the long-run implications of fiscal policy include Foley and Sidrauski (1971), Blinder and Robert Solow (1974) and Tobin and Buiter (1976). Let us follow Blinder and Solow (1973) for a moment. Recall that according to the old short-run story, a deficit-financed increase in government expenditure implies that the IS curve shifts to the right substantially and, via a "portfolio effect", the LM curve shifts slightly to the left so that both output and interest rates rise. What happens in the next period? As the deficit remains in the next period (recall, it is a flow), there will be another increase in bond supply which will unbalance people's portfolios again - thus, once again, the resulting excess supply of bonds and excess demand for money will require a higher rate of interest to restore short-run equilibrium. Thus, in this period, there is another shift in the LM due to the new bond issue. As long as the deficit remains, this will happen every period. We can see that a problem quickly emerges here: as the LM shifts left, income falls and as income falls, tax receipts decline so that the deficit actually widens. This would, in turn, require ever-increasing bond issues by the government with ever-increasing rates of interest - even though government expenditure remains the same. In time, not only would private investment be completely crowded out, but output would actually collapse to zero while the rate of interest approached astronomical heights! This unstable result was quite unsettling. Blinder and Solow (1973) attempted to circumvent this by noting that as government bonds and money constitute "net wealth", then, by the wealth (Pigou) effect, consumption and hence aggregate demand would rise as the stock of bonds rose. Thus a bond-financed deficit would also engender a rightward shift in the IS curve every period which would counteract the fall in output from the leftward shift in the LM curve. The next question is obvious: which effect is stronger and will the process converge? Leftward shifts in LM due to the portfolio effect and rightward shifts in IS due to the Pigou effect will lead to higher and higher interest rates for sure, but the net effect on output is unclear. Blinder and Solow posited that the wealth effect is stronger than the portfolio effect so that, on net, output will rise and, as tax revenues increase with income. In this case, the we would eventually converge to a balanced budget - as Christ (1968) had insinuated. However, at this long-run equilibrium, both output and interest rates would be higher. As noted, this convergence depends crucially on assuming that the wealth effect is considerably stronger than the portfolio effect. This may not be very credible for a variety of reasons. Firstly, as the real balances debate revealed, the empirical importance of the Pigou Effect is bound to be very small. Secondly, debt-service payments (i.e. interest payments on the debt) will increase consumer income (and thus consumption) and reinforce the debt (and, via the wealth effect, consumption again). Thus, if the wealth effect is strong and debt-service is expensive, then the interest payment may induce ever-greater consumption-cum-output expansions and possibly even instability if tax revenues do not rise as fast as debt servicing costs. In this case, the economy seems to be able to borrow and spend itself into heaven in the long-run. Blinder and Solow (1973) nonetheless ruled out this possibility - albeit with somewhat ad hoc (and outlandish) assumptions about the magnitude of the effects and the imposition of the condition that the interest rate not exceed the growth of tax revenues. However, although Blinder and Solow argued that there is not complete "crowding out" (otherwise there could not be convergence), other economists have argued, on the basis of these models, that this is in fact possible. The "crowding-out" effect of a money-financed increase in government spending would be much smaller and even non-existent in the long-run. The idea is simply that a money-financed expansion will lead to both an increase in interest rates (due to a rise in money demand from greater output) and a decrease in interest rates (from higher money supply). The net effect may be, in the short run, a higher interest rate and thus some degree of crowding out via the money demand effect, but, in the long-run, as the deficit is carried over, there will be further issuing of money and the interest rate will continue to climb down and raise output until enough is generated to yield the taxes necessary to finance the initial spurt of government spending. Thus, in the end, the interest will be the same as it started. It is interesting to note that the relatively quick convergence of this process implies that it is entirely possible that a money-financed fiscal expansion will lead to a smaller increase in output in the long-run than a bond-financed fiscal expansion. This is particularly surprising as precisely the opposite is the case in the short-run. Finally, one should mention some of the public finance concerns of more "growth-oriented" theories. In the simplest Keynsian growth theories (e.g. Domar, 1944), some form of an accelerator mechanism to investment can be added to the basic Keynesian model so that bond-financed increases in government spending induce further private investment. This is a form of "real crowding in". The idea is that investment increases if expected goods demand rises and that past changes in output are used as predictors by firms' of future demand. Thus, as output rises from government spending, this accelerator mechanism implies that firms expect higher demand and thus will increase investment. A more short-run version of "real crowding in" would be that the rising output induced by a bond-financed fiscal expansion could lead to expectations of higher future profits and thus increasing valuation of stock prices (raising Tobin's q), thereby reducing their required rate of return and consequently inducing greater private investment (e.g. Tobin, 1969; Blanchard, 1981). However, in a more Neoclassically-minded growth context, there is still some room for "crowding out" arguments which invoke the spector of the old "burden-of-debt" fears. Specifically, it has been argued (e.g. Modigliani, 1961) that government bonds will replace capital (i.e. equity) in people's asset portfolios and that, with less capital, there will be a smaller basis for future growth. However, if government spending is mostly in capital projects and infrastructure, this argument is neutralized as capital-formation ensues. Furthermore, there may very well be "supply-side" effects of government spending e.g. changes in labor supply incentives, infrastructure effects, etc., which can make private investment even more profitable and thus lead to higher capital formation. Abba Lerner (1943, 1944, 1973), Heilbroner and Bernstein (1989) and many other economists have argued along these "crowding in" lines. (C) The Ricardian Equivalence Hypothesis An interesting development that emerged in the 1970s and has since been associated with New Classical theory but is still relevant to our context, was the proposition of the "Ricardian Equivalence Hypothesis" by Robert Barro (1974). In order to combat the peculiar Blinder-Solow results, Barro sought to prove that the Pigou Effect was inoperative for the debt-financed case by arguing that government bonds were not "net wealth". Under a particular set of assumptions (e.g. intergenerational altruism or immortality, perfect capital markets, lump sum taxation, and the condition that debt not grow faster than the economy), Barro argued that every bond-financed deficit must be met by a future tax increase, that this tax increase would be foreseen by living agents and that these agents would care enough about posterity to adjust their present consumption accordingly. In short, this implies that agents do not take a bond-financed increase in government spending as a lucky windfall but will save the entire proceeds in anticipation of the future tax burden - and thus not raise their demand for goods and services. The implication of the Ricardian Equivalence Hypothesis (henceforth, "REH") is that interest rates and consumption will be unaffected by debt-financed government spending. From a Neoclassical "loanable funds" view, REH argues that the rise in the demand for loanable funds (government deficit) will be met by an exactly equivalent rise in the supply of loanable funds (savings in anticipation of future taxes) - thus interest rates remain unchanged. Equivalently, in a Keynesian framework, the REH implies all the income arising from the debt-financed government expenditure will be saved into the perfect hedge - the newly-issued government bonds themselves - so that consumption does not increase and thus the multiplier is killed off. However, one should be careful to distinguish the implications of this "debt-neutrality" argument. While the Ricardian Equivalence Hypothesis implies that interest rates should not rise in response to increases in government debt, they do allow for the argument that interest rises in response to increases in government spending. This last effect is related to the assumption of full employment in the New Classical mode being combined with Ricardian Equivalence. The impact of the Ricardian Equivalence Hypothesis has been twofold: firstly, it has generated a lot of literature (particularly by the so-called New Keynesians) on themes which yield "anti-Ricardian" results. However, acceptance of REH does not imply that government fiscal policy does not "matter". Indeed, Robert Barro has argued time and again that the form of government spending and taxation should be examined for potential real implications on the economy in terms of the profile of goods and services produced. The different implications of permanent and temporary changes of government spending on interest rates and the theory of "optimal taxation" is an example of this type of work. One surprising outcome of REH is that it seems to provide a Neoclassical version of the Keynesian "functional finance" theories of Abba Lerner - albeit minus the multiplier! It is surprising to find, towards the turn of the century, Neoclassical economists such as Robert Barro (1981) and D.A. Aschauer (1989), analyzing fiscal policy according to optimality and efficiency (and, potentially, equity) of government expenditure while holding on to the notion of debt-neutrality. They have many times argued for increased government roles in public projects to "crowd in" private sector output and improve allocation - in short, for conducting a Neoclassical version of Lernerian "functional finance"! In a spectacular contrast, the "New Keynesians", self-declared inheritors of the Keynesian mantle, are in the meantime busily constructing arguments to disable Ricardian Equivalence and thus support their contention that not only do government deficits "crowd out" private investment, but the entire "buden-of-debt" rhetoric re-emerges. As one powerful and prominent New Keynesian has put it:
So, as the New Keynesians argue along these old pre-Keynesian lines, the modern Neoclassicals are busy thinking about .... functional finance? It's a topsy-turvy world we live in!
|
All rights reserved, Gonšalo L. Fonseca