|
Contents There are several important relationships within the Keynesian model: the consumption function, the investment function, the liquidity preference function being the major three. Methodologically speaking, the Neoclassical-Keynesian Synthesis had given the green light for the application of Neoclassical economic theory to Keynesian economics and thus major efforts were undertaken by various Neo-Keynesians to "ground" these major Keynesian relationships in Neoclassical microeconomic theory - specifically, to derive these relationships from utility-maximization or profit-maximization exercises of some sort or other. This turned out to be perhaps the major research agenda in American macroeconomics during the 1950s and 1960s and only a brief outline is possible here. (A) The Consumption Function The first efforts in this research programme concentrated on the consumption function. The simple Keynesian function, C = C0 + cY was regarded as "too simple" and there were apparently some conflicts with empirical evidence laid out by Simon Kuznets (1946) - specifically, the finding that savings were a reasonably stable share of income for the 1869-1938 period. As the simple Keynesian consumption function predicted that as income rose, savings would take an ever greater share of it, efforts were needed to come up with a "better" consumption function. James S. Duesenberry (1949) mad the first attempt, by arguing that there was "habit formation" in consumption behavior. Specifically, he proposed that people easily increase consumption when income rises but have problems reducing it symmetrically when income falls. Thus, he proposed a consumption function of the form:
where YM is the peak consumption achieved in the past. In this way, consumer behavior is "ratcheted up" as income rises above previous peaks. Franco Modigliani and Richard Brumberg (1954) decided to go further than Duesenberry and proposed what has since become known as the "Life Cycle Hypothesis" (LCH). A similar idea was proposed independently by Milton Friedman (1957) in his "Permanent Income Hypothesis" (PIH). Specifically, Modigliani and Brumberg visualized consumption decisions as integrated in an intertemporal optimization program for a "representative consumer" along the lines of Irving Fisher's (1930) two-period model. In other words, households were faced with a forecasted "income stream" which they attempted to allocate over their lifetime. They did so by shifting their promised endowments around different time periods via financial markets in order to "smooth" their consumption out (or, rather, to make it compatible with their intertemporal tastes). Their optimization problem can be roughly stated to be something akin to the following (we are summing time t from t = 0 to t = T, the end of one's life):
thus agents choose a consumption path (C1, .., CT) which maximizes their intertemporal utility function (weighted by a subjective time discount rate b t) subject to an intertemporal budget constraint which is governed by a discounted stream of future income (å t Yt/(1-rt)) and the wealth they are born with (A0). Modigliani and Brumberg proposed an aggregate consumption function of the following sort:
where Vt is the sum of net worth at time t, income at time t and the present value of all expected future earnings - all averaged out over the characteristics of the given population. In Miton Friedman's (1957) language, Vt can be thought of as "permanent income". The parameter c can be assumed constant if the demographic composition of the population is relatively constant. Thus, in short, the aggregate consumption function does not depend solely on current income, but also on demographics, on conditions in financial markets (and thus the interest rate), uncertainty and expectations, etc. To capture this, Ando and Modigliani (1963) proposed the following testable consumption function:
where consumption at time t is a linear function of current income (Yt), current wealth (At) and the average expected annual non-property income (T-1)Yte. This last term is obtained by recognizing that (T-1)Yte = å tYt/(1+r)t and is the most difficult part of this equation to estimate. The implication, then, is that temporary increases in income will not have much of an effect of consumption behavior but rather be accumulated as savings, whereas changes in the expected income stream (permanent income) will lead to substantial changes in consumption. (B) The Investment Function Keynes's theory of investment was a more complicated affair. Unlike consumption, the modifications of the investment function were not dominated by empirical concerns - indeed, empirical evidence seemed to imply that interest rates has, in fact, little influence on investment (hence many Neo-Keynesian economists were happy enough to assume a vertical IS curve which permitted them to more-or-less ignore the LM side of things in the early years). From this vantage point, the effort should have been to integrate the empirically-powerful accelerator theory of investment into the Keynesian model - as was indeed attempted by Roy Harrod, Evsey Domar and the Cambridge Keynesians. However, the American Neo-Keynesians were more worried about the theoretical problems of the simple Keynesian investment function (see our notes on investment for more details). In fairness, integrating investment (a flow concept) with a stock-based capital and production theory had already been a troublesome issue for Neoclassical theory. Recall that Keynes proposed an investment function of the sort I = I0 + I(r) where the relationship between investment and interest rate was of a rather naive form: namely, firms "ranked" investment projects depending on their internal rate of return (or "marginal efficiency of investment") and thereby, faced with a given rate of interest, chose those whose rate of return exceeded the rate of interest. Assuming something akin to a continuum of available investment projects, they would thus invest until their marginal efficiency of investment was equal to the rate of interest, i.e. MEI = r. Several problems emerged with this theory. Firstly, as Armen Alchian (1955) and, later, Jack Hirshleifer (1970), pointed out, the method by which Keynes "ranked" projects, i.e. via their "internal rate of return", implied that Keynesian rankings are ultimately quite different from rankings which are set according to the maximization of the present value of firms. A second objection, perhaps best expressed by Robert Eisner and R.H. Strotz (1963) was regarding the rather ad hoc way Keynes dealt with the determination of the expected profits and returns, arguing instead for some carefully prescribed distribution and process of expectation formation. This, of course, is a "Neoclassical" objection - Post Keynesians, such as G.L.S. Shackle (1949, 1955) and Paul Davidson (1972, 1994) have vociferously insisted that there is no ad hoccery at all. Quite the contrary - pointing to Keynes's (1936, 1937) repeated insistence on radical uncertainty - indeterminate or "animal spirited" expectations are quite essential to Keynes's theory of investment. A third, perhaps more serious problem, was the contention that a downward-sloping aggregate MEI curve is ultimately incompatible with Keynes assumption of unemployment (e.g. Piero Garegnani, 1978).. In situations of unemployment, increases in investment will increase effective demand and thus the profitability of projects - which will thereby push the MEI outwards. Consequently, as long as there is unemployment, the MEI curve should in fact be flat. Salvaging the downward sloping MEI curve may only be possible by either careful considerations of aggregation problems or appealing to Kalecki's (1937) principle of increasing risk - which again, coincides with the uncertainty thesis in Keynes' (1936, 1937) and the instrumental importance of finance. Jack Hirshleifer (1958, 1970) had expanded upon Fisher (1930) to argue that investment ought to be conceived in the context of the maximization of the present value of the firm. Subsequently Dale W. Jorgensen (1963, 1971) provided a micro-theoretic Neoclassical theory of "investment" based on this notion. Effectively, Jorgensen's solution was to steal a leaf from the consumption book and recast the optimal capital stock decision in intertemporal form. Firms attempted to choose an intertemporal path for capital stock that maximized the present value of the firm - i.e. the present value of a stream of proceeds. Thus, in general, firm's face the following intertemporal optimization problem:
where (suppressing time subcripts) they are maximizing a stream of returns (defined as total sale revenue, pY minus wage costs, wL and investment costs, sI where s is defined as the supply price of investment) subject to a production function constraint, Y = F(K, N) and the definition of net investment, dK/dt. Solving this problem yields the following conclusions: (1) FN = w/p, firms employ labor until their marginal product is equal to their wage; and (2) the following holds:
so that the optimal capital stock K* is chosen where the marginal value product, pFK is equal to the real user cost of capital, c = s[d + r - (ds/dt)/s]. This last term can be thought of as the implicit rental rate. The logic is that the cost of investing (i.e. buying another unit of capital) is the opportunity cost of lending out the funds (r), the depreciation per unit (d ) minus the expected capital gains (ds/dt)/s. If we had an explicit invertible production function, then K* could be determined easily from FK = c/p. For instance, suppose we had a Cobb-Douglas production function Y = Ka L(1-a ) so that FK = a (Y/K), then K* = pa Y/c. Thus, in general, K* = ¦ (Y, p, r, d , s, ds/dt, p) or simply K* = ¦ (Y, p, c) where K* depends positively on Y and p and negatively on c. This is fine for determining the optimal capital stock, but how does one solve for the investment flow? Investment is defined as the instantaneous change in the optimal stock of capital, thus, in principle, there is no investment unless there is some reason to change the optimal stock of capital (by say, imposing exogenous some rate of technical change or some population growth rate), or, alternatively, investment is derived from the adjustment path towards the optimal capital stock, K*. Following the first case, suppose that Kt* ¹ Kt+1* for some reason. Then, in principle, moving to continuous time, from any given K, then investment is defined as I = dK* + d K, thus:
thus investment is a function of changes in the real user cost of capital (c), changes in the price of output (p), changes in output (Y) and the level of capital (K). Jorgensen's subsequent addition of some controversial delivery lags has been since disputed and thus will be ignored here. Of course, Jorgensen's (1963) theory is less about investment and more about optimal capital. If investment is seen as the adjustment from a given level of capital to the optimal level of capital stock, then, in Jorgensen, investment is instantaneous - and thus Jorgensen's connection with Keynes is tenuous at best. As Abba Lerner (1944, 1953) and Trygve Haavelmo (1960) pointed out, it is virtually impossible to allow marginal productivity theory to determine the "optimal" level of capital, and then have marginal efficiency of investment theory determine the optimal level of investment without thereby eliminating the flow investment term entirely. One implied resolution was to appeal to marginal adjustment costs. In effect, rising supply price of capital goods industries implies that firms approach the optimal capital stock only gradually. This gradualness is governed by marginal adjustment costs which are, in turn, the reason for a falling marginal efficiency of investment (MEI) function. Thus, as investment increases, supply price of capital goods rises and thus MEI falls so that MEI = r before the optimal capital stock is reached. These marginal adjustment costs will therefore slow down adjustment and allow for both optimal capital and optimal investment to be defined. Attempts were made to incorporate marginal adjustment costs into Jorgensen's theory in order to obtain a proper theory of investment in an optimization context. To this end, the work of Robert Eisner and Robert H. Strotz (1963), Robert E. Lucas (1967) and John P.Gould (1968), was instrumental. Specifically, instead of the term sI in Jorgensen's equation, the Eisner-Strotz-Lucas-Gould modification was to propose sI + C(I)sI where C(I) is a convex function reflecting marginal adjustment costs. These adjustment costs can be due to "intrinsic" factors (i.e. costs of installation) or "extrinsic" factors (rising supply price). Thus, firms now face the problem:
which, solving via standard dynamic optimization techniques, yields a pair of differential equations:
where, if we had explicit terms for C(I) we could solve for the path K(t) and q(t). Thus, the marginal adjustment cost model does not yield an "optimal capital" level but rather an optimal adjustment path. The q defined in the first equation is actually James Tobin's "q" (which, in this model, is defined as q = l /s where l is a costate variable representing the shadow value of capital). Notice that in our net investment equation, dK/dt, we have the implicit function y (q) - i.e. a Keynesian "investment" schedule y (q) where y (1) = 0 and y ¢ > 0. James Tobin's "q" theory of investment was presented in Brainard and Tobin (1968) and Tobin (1969). Effectively, Tobin's q theory proposes that a firm will invest until q = 1 where q is defined as the ratio between the stock-market valuation of existing real capital assets and its current replacement cost. In Keynes's (1936: p.135) language, q = V/C where V is what Keynes defined as present value of the prospective yield of the capital asset while C is what he defined as the supply price of the capital asset. Consequently, at the margin, q can be seen as the ratio of the marginal efficiency of investment to the rate of interest, i.e. q = MEI/r so that the Keynesian investment function can be rewritten as I(q - 1) where firms invest until q = 1 (or, equivalently, MEI = r). As we can see immediately from above, this function is captured by y (q). Thus, as other commentators have noted, the Eisner-Strotz-Lucas-Gould theory of investment with marginal adjustment costs is formally equivalent to Tobin's "q" theory of investment - and, of course, logically equivalent to what Abba Lerner (1944, 1953) had already long proposed. Notice, finally, the main difference between the adjustment cost story and the Jorgensen one regarding the rate of interest: if r rises, q falls and consequently investment collapses in the adjustment cost story - just as Keynes proposed; in Jorgensen, bar the contrivance of ad hoc lags, optimal capital stock falls but investment just "jumps". (C) The Liquidity Preference Function One of the early items to be tackled was Keynes's money demand function. Before the General Theory, John Hicks (1935) had explained that money demand ought to be analogous to any exercise of choice by the consumer - in this case, choice of portfolio holdings, and that therefore the principles of marginalist economics ought to be applicable to determining optimal money holdings (and those of other assets). In Hicks's view, the opportunity cost of money was the interest foregone on other assets. This concept, already insinuated by Keynes in his Treatise on Money (1930) was enshrined in and expanded upon in the "liquidity preference" theory of interest of Keynes's General Theory (1936). Keynes (1936) designated three motives for holding money: for transactions purposes (to finance regular expenditures); precautionary purposes (to finance unexpected expenditures); and speculative purposes (to hold as an asset). In terms of Keynes' liquidity preference function, money demand was affected by current income and interest rates so:
where r is bond interest rate (an average of rates of returns on illiquid assets) and Y is income. The basic proposition was that Lr < 0 and LY > 0 - although a specification more faithful to Keynes's original system would include expected and current interest rates into this function. Keynes originally proposed that interest would only affect speculative demand - by far, the most controversial of the three motives. Specifically, the basic problem is that under normal circumstances, money pays no interest whereas other assets (such as bonds) do. Since bonds are essentially promises to pay a certain amount of money at a future date (plus interest), then there seems to be no reason why one should hold money as an "asset" instead. Keynes's original answer revolved around the issue of expectations. If, for instance, an agent expects interest rates to rise in the future, then to avoid expected capital losses, he will hold his wealth in money and await for that rise. Once it has risen, he can then purchase bonds. The inverse of this analysis applies to an agent who expects interest rates to fall: she would buy bonds now rather than later. When interest rates collapse and bond prices rise, she can then sell her bond and make capital gains. Thus, Keynes treated his agents as "plungers", i.e. in other words, they will hold all of their wealth in money or all of their wealth in bonds, depending upon their expectations of interest rate movements. In other words, in terms of the speculative demand for money, an agent does not have a diversified portfolio. Consequently, for there to be an "aggregate" portfolio which includes both money and bonds, then there necessarily must be heterogeneity of expectations. It must not be that everyone expects interest rates to increase or else no one will hold bonds and everyone will try to hold money (notice that Hicks's (1937) "liquidity trap" would be precisely such a case). If an agent is willing to hold money, then somebody else must be willing to hold bonds - and to explain this, Keynes argued, their expectations must differ. For Neoclassical economists used to arguing on the basis of a single "representative" agents with relatively rational (and thus relatively homogeneous) expectations, Keynes's explanation for the relationship between interest and money demand - via a world of heterogeneous agents with differing (and thus irrational?) expectations - must have sounded like a sour note. Instead, they looked upon Hicks (1935) to offer them a way of maintaining a relationship between money demand and interest without Keynes's "speculative demand". The first set of propositions was the reformulation of the "transactions demand for money" as an optimization problem by William Baumol (1952) and James Tobin (1956). Specifically, they proposed that people needed money to undertake transactions (Y), but if they hold too much money, they sacrifice the opportunity cost of holding bonds (the interest rate r). However, if they do not hold enough money, they have to convert bonds to money several times over during any transaction period (n times per period) and thus incur transactions costs (c per conversion). Thus, suppose that W is the amount an agent converts from bonds to money every time he converts. Assume he receives some income (Y) over a period and that he spends all his income, thus must convert it all. Assuming he converts n times, then nW = Y. Thus, over the period, average money holdings are M = W/2 and average bond holdings are the remainder, i.e. B = (Y -W)/2. If it costs him c every time, then total transactions costs are C = cn. Assume, of course, that rB > C so that it is always worthwhile to hold bonds. The consumer's decision to hold money can be expressed as the solution to the following optimization problem:
which, plugging everything in and solving, yields the following:
which is the famous Baumol-Tobin "square root rule" for the optimal transactions demand for money. Notice, then, that money demand M* is positively related with income and negatively related with the rate of interest on bonds - thus the relationships within the regular money demand function, L(r, Y) can be explained solely via this "transactions" motive. The precautionary motive for holding money was also formalized as an optimization problem - this time by E.J. Whalen (1966) and M.H. Miller and D. Orr (1966). Their basic point was that people hold money to finance unexpected purchases. Consequently, these economists decided to formalize precautionary demand by having net unplanned disbursements be some random variable (distributed around zero with finite variance). Transactions costs and interest rates are also included in the story so agents have an incentive not to hold everything in money (thus interest rates) and not to hold everything in bonds (thus transactions costs). Following an effectively similar procedure to Baumol-Tobin, we obtain the following:
a "cube-root" rule which relates precautionary money demand (M*) positively to transactions costs and the variance of net disbursements (uncertainty) and negatively to the rate of interest. The most celebrated liquidity preference function was the attempt to formalize the speculative demand for money (as an "asset") by James Tobin in his famous article "Liquidity Preference as Behavior Towards Risk" (1958). Together with Harry Markowitz (1952), Tobin's (1958) article effectively launched "modern portfolio theory" and, consequently, much of the rest of modern finance theory. Tobin's principle was simple: people are faced with a variety of assets with higher return and higher risk than money. If people are also risk averse, they may still wish to hold riskless money in spite of the fact that it has zero return. Tobin's more interesting result was than, in constructing a portfolio that maximizes expected utility, people will hold both bonds and money in their optimal portfolio. This, of course, is based on the simple principle of diversification. To see this in a simple scenario, suppose we have two assets, bonds and money. The return on bonds is merely RB = r + G where r is the interest rate and G are capital gains. Capital gains on bonds are assumed to be distributed with mean zero (E(G) = 0) and finite variance (var(G) = s G2). Thus, the expected return on the bond is merely E(RB) = r. Money is riskless and returnless, so RM = 0. In the mean-variance (or rather mean-standard deviation) space, as in Figure 6, we can denote the return-risk positions of a unit of money at the origin 0 and a unit of the bond at point B. A "portfolio" consists of a bundle of bonds and money, thus the "return" on a portfolio, Rp is merely a linear combination of the individual returns (RB, RM) with the weighting parameter a denoting the relative proportion of the portfolio dedicated to the specific asset, i.e. Rp = aRB + (1-a)RM = aRB as RM = 0. The expected return on the portfolio, thus, is E(Rp) = aE(RB) = ar. The "risk" of the portfolio, however, is:
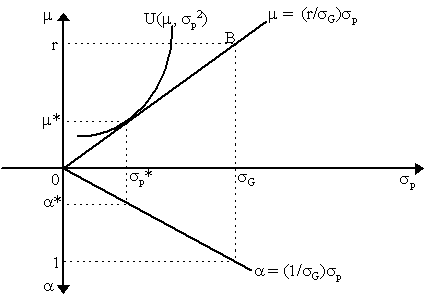
i.e. it is related to the variance of the capital gains. Consequently, we see that we can write the portfolio weight as a = sp/sG. Thus, the expected return on the portfolio can be rewritten as follows E(Rp) = ar = (r/sG)sp, i.e. the expected return on the portfolio is some linear function of its standard deviation. We can draw this line in Figure 6 (m = E(RP)) and it represents the "opportunity locus", or the various combinations of portfolio risk and expected return available - the line connecting the origin to point B with slope r/sG.
Notice that once we obtain sp, we can immediately obtain a by appealing to our old relationship that a = (1/sG)sp. This is shown in Figure 6 in the lower quadrant as the line extending from the origin with slope (1/s G). Thus, for any combination of risk and return (m , sp2) in the opportunity locus in the upper quadrant, we can, bouncing off the line in the lower quadrant, obtain the implied portfolio allocation decision, a . All that remains to be determined is the "optimal" portfolio allocation a *. For this, we need an expected utility function in mean-variance space, i.e. some U = U(m , sp2) which is positively related to expected return and, by risk aversion, negatively related to variance. As shown in Figure 6, assuming convexity, continuity and other nice properties for utility, then maximizing U(m , sp2) subject to the opportunity locus m = (r/sG)sp, we obtain the optimal portfolio risk-return combination (m*, sp*) which, bouncing off the locus in the lower quadrant, yields the optimal portfolio allocation decision a *. Notice that the optimal decision is at neither extreme - thus, at the optimal portfolio, people hold both money and bonds. The negative relationship between money holdings and interest rates is easily visualized in Figure 6: simply if r rises, swing the opportunity locus anticlockwise and we obtain a new optimal portfolio allocation - which, assuming the substitution effect dominates, will be higher than the old a* - thus people reallocate their portfolios such that they increase their bond holdings relative to money - thus the interest rates and money demand are negatively related when money is treated as an asset. Thus, risk-aversion, as Tobin (1958) argued, can be a rationalization of the "speculative demand" for money. (D) The Transmission Mechanism Keynes (1936) had broken the Neoclassical dichotomy between monetary and real phenomenon in a decisive fashion via his liquidity preference theory of interest. However, following developments in integrating various Keynesian relationships with Neoclassical microeconomic logic, the traditional channels by which monetary phenomena (money, bonds, interest, etc.) were "transmitted" to the real economy (output, employment, etc.) were modified and expanded. No longer was it simply that, say, increases in the supply of money led to declines in interest and thus rises in investment. Things could be more complicated and operate through various channels. As Franco Modigliani (1971) stresses, one of the new avenues of transmission was consumption. The Life Cycle Hypothesis outlined earlier made consumption also a function of interest. How a particular agent's consumption would react in response to, say, a rise in the interest rate, however, depends on the relative power of various effects: the substitution effect implies consumption will fall, the income effect implies it will rise (if a lender) or fall (if a borrower) and the wealth effect implies it will fall. Thus, the standard assumption is that a general consumption function C = C(r, Y) would be negatively related to interest. The impact of financial factors on investment seemed clear enough by Keynes's MEI rule. However, James Tobin (1969) worked out some more details. Specifically, a rise in the bond interest rate can lead directly to higher cost of capital and thus lower investment (the standard channel). However, the rise in interest rate can lead to lower valuation of current assets - namely, decreasing Tobin's "q" and consequently investment. Furthermore, if firms use capital as collateral on bank loans, lower valuation on the financial markets implies less valuable assets and thus potentially higher borrowing costs. This is similar to some of the discussions on the "credit" channel of monetary transmission particularly pertinent to New Keynesian stories (thus we shall postpone our discussion of this). For an example of the richer relationship between financial factors and output levels, see Olivier Blanchard's (1981) integration of Tobin's q into a simple IS-LM model. Mention should also be made at this point of the work of Robert Mundell (1962, 1963, 1968) and J.M. Fleming (1962) in extending of the IS-LM apparatus to incorporate a foreign sector, which will be analyzed later. This not only expanded the Keynesian portfolio to include foreign assets, but also incorporated new variables, such as the exchange rates, into the model which have become, in turn, incorporated into standard transmission mechanism. Finally, one important set of innovations was the enrichment and integration of the IS and LM constraints. Recall that Keynes had posited his own dichotomy, dividing the consumption-savings decision of the household from the simple money-bonds portfolio allocation decision. Lloyd Metzler (1951), J. Gurley and E.S. Shaw (1960), James Tobin (1961, 1969) and W. Brainard and J. Tobin (1963, 1968) greatly expanded upon this by increasing the asset menu available to households to include short-term bonds, long-term bonds, equity, financial intermediaries and bank loans, foreign assets, currency, etc. in their portfolio decision. In this case, excess money supply no longer feeds "immediately" and necessarily into excess demand for bonds, but rather could feed into greater demand for a greater variety of assets, even implying that some categories of interest rates might fall. By enriching the simple Keynesian portfolio, the transmission mechanism becomes more complex, but useful insights, for instance about term structure, effects of monetary policy, crowding out, etc. were obtainable. Some economists, notably Milton Friedman (1956) and Sho-Chieh Tsiang (1956, 1966, 1980, 1982) took this a step further and suggested that the IS and LM constraints should be integrated with each other. Specifically, they disputed the strict "Tobinesque" separation of the consumption-savings decision from the portfolio allocation decision - a point of contention that harked back to the Keynes-Robertson-Ohlin debate of 1937. What Friedman and Tsiang suggested, and was only later taken up by James Tobin (1982), was that instead of a multiplier flow constraint (I-S) = 0 being accompanied by a self-contained wealth constraint, (Md - Ms) + (Bd - Bs) = 0, one ought to include the "flow terms" of savings (demand for wealth) and investment (supply of wealth) as potential equilibrators of the portfolio decision. In other words, they envisaged the following sort of generalized Walras's Law constraint for stock variables:
where D Wd and D Ws are changes in the demand and supply of wealth respectively. As we can conceive of savings as a change in the demand for wealth and investment as a change in the supply of wealth, then DWd - DWs = S - I and so the new portfolio constraint becomes:
where we now have the flow variables of the goods market decision in the portfolio stock constraint. Thus, it is now possible that, say, an increase in money demand does not necessarily imply a reduction in bond supply (as was necessarily implied before) but it can lead to an increase in savings instead. In other words, the portfolio decision spills over into the flow decisions. Milton Friedman's (1956) transmission mechanism suggested precisely this kind of integration of flow and stock constraints: excess money supply, he claimed, might leave bond interest rates unchanged and spill over directly into excess demand for goods, i.e. Md - Ms < 0, Bd - Bs = 0 and I - S > 0, and that will lead, by the multiplier, to an increase in output - thus the "money-income" causality debate that Friedman sparked. In his Nobel lecture, James Tobin (1982) provided an enriched multi-asset IS-LM model with overlapping stock-flow decisions precisely of this type.
|
All rights reserved, Gonçalo L. Fonseca